 |
AdvDiffusionFemPhysics
An advection-diffusion equation FEM Physics Plugin
|
 |
AdvDiffusionFemPhysics
An advection-diffusion equation FEM Physics Plugin
|
The classical dual mixed finite element method for flow simulations is based on \(H(\text{div},\Omega)\) conforming approximation spaces for the flux, which guarantees continuous normal components on element interfaces, and discontinuous approximations in \(L^2(\Omega)\) for the pressure. However, stability and convergence can only be obtained for compatible approximation spaces. Stability finite element methods may provide an alternative stable procedure to avoid this kind of delicate balance. In this GeMA FEM Physics was implemented a high-order finite element methodology to solve the Darcy problem based on the combination of an unconditionally stable mixed finite element method with a hierarchical methodology for the construction of finite dimensional spaces of \(H(\text{div},\Omega)\) and \(H^1(\Omega)\).
Consider a unsteady version of Darcy problem, for a given finite time \(t_f>0\), consider the time evolution problem: Find the velocity \(\mathbf{u}\) and the the pressure \(p\) such that
\begin{eqnarray*} \mathbf{u} = -\mathbf{K}\,\text{grad}(p) & \text{in }\Omega & (\text{Darcy's Law})\\ \phi \, \partial_t p + \text{div}(\mathbf{u}) = f & \text{in }\Omega & (\text{Mass Conservation})\\ p = p_d & \text{in }\partial \Omega_D & (\text{Boundary Condition for Pressure})\\ \mathbf{u} = \mathbf{u}_n & \text{in }\partial \Omega_N & (\text{Boundary Condition for Flux})\\ p(\cdot , t = 0) = p_0 & \text{in } \Omega & \text{(Initial Condition)} \end{eqnarray*}
where \(\Omega \subset R^n\) ( \(n=1,2,3\)) is an open bounded domain with Lipschitz boundary \(\Gamma = \partial \Omega\) representing the heterogeneous porous medium, \(f\in L^2(\Omega)\) is a distributed source/sink function and \(\mathbf{K}\) is a symmetric positive-defined tensor representing the permeability divided by the fluid viscosity and \(\phi\) the porosity of the porous matrix.
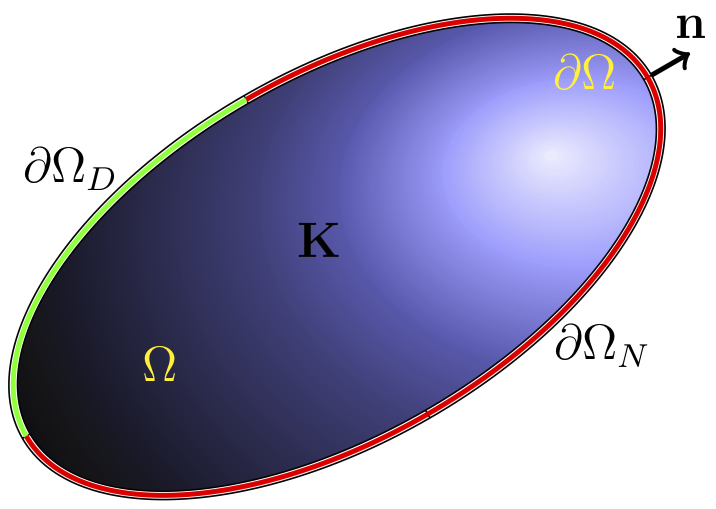
Using a notation and concepts show in Hierarchical high order finite element spaces in \(H(\text{div},\Omega) \times H^1(\Omega)\) for stabilized mixed formulation of Darcy problem , the variational form for steady Darcy's problem with homogeneous boundary conditions for pressure is:
Find \(\{\mathbf{u},p\}\in \mathbf{U}_{l}\times \mathbf P_{k}\) such that
\[ A((\mathbf{u},p);(\mathbf{v},q)) = F(\mathbf{v},q) \quad \forall (\mathbf{v},q)\in \mathbf{U}_{l}\times \mathbf P_{k} \]
where
\begin{eqnarray*} A((\mathbf{u},p);(\mathbf{v},q)) = (\mathbf{\Lambda}\mathbf{u},\mathbf{v}) - (\text{div}(\mathbf u) , p) - (\text{div} (\mathbf{v}),q ) + \frac12(\text{div}(\mathbf{u}),\text{div}(\mathbf{v})) - \frac12 (\mathbf{K}(\mathbf{\Lambda}\mathbf u + \text{grad}(p)),\mathbf{\Lambda}\mathbf v + \text{grad}(q))\,, \end{eqnarray*}
\[ F(\mathbf{v},q) = -(f,q) +\frac12(f,\text{div}(\mathbf{v})) \]
considering \(\mathbf{\Lambda} = \mathbf{K}^{-1}\) is well defined, and assume that we take finite-dimensional subspaces \(\mathbf{U}_l \subset H(\text{div},\Omega)\) and \(\mathbf{P}_k\subset H^1(\Omega)\).
For a construction of \(H^1(\Omega)\) and \(H(\text{div}, \Omega)\) conforming subspaces, let \(T_h\) be a regular family decomposition of \(\Omega\) into regular quadrilateral elements \(e\) such that \(\bigcup _{e \in T_h} e = \Omega_h \subset \Omega \). A regular master element \(\hat{e}\subset R^{n}\) and \(F_e\) a smooth mapping of \(R^n\) into \(R^n\) such that, we defined \(e = F_e(\hat{e})\) for all \(e \in T_h\). We suppose that the Jacobian matrix \(DF_e(\mathbf{\xi})\) is invertible for any \(\mathbf{\xi}\) and that \(F\) is globally invertible on \(e\). We then have
\[ DF_e^{-1}(\mathbf{x}) = \left(DF_e(\mathbf{\xi})\right)^{-1}, \]
and for \(\mathbf{\hat{\mathbf \phi}}\in \left(L^2(\hat{e})\right)^n\), the mapping
\[ G_e(\mathbf{\hat{\phi}})(x):= \frac{1}{|\text{det}\,DF_e({\xi})|} DF_e(\mathbf{\xi})\mathbf{\hat{\phi}}(\mathbf{\xi}), \quad \xi = F_e^{-1}(x) \]
know as Piola's Transformation . With this geometrical considerations, we defined the trial finite element subspaces how
\[ \mathbf{U}_l = \{\mathbf{v} \in H(\text{div},\Omega_h); \mathbf{v}|_e = G_e(\hat{\mathbf{v}})(x) \, , \hat{\mathbf{v}}\in \mathbf{Q}_l(\hat{e} ) \, , \forall \, e \in T_h\} \, ,\qquad \mathbf{P}_k = \{ q \in H^1(\Omega_h) ; \, q|_{e} = (\hat{q} \circ F^{-1}_e)(x) \, , \hat{q} \in Q_k(\hat e)\, , \forall e \in T_h \}\, . \]
Used definitions of hierarchical and conformity elements show in Higher-order finite element methods we construct the local \({Q_k(\hat{e})}\) conforming finite subspace of degree \(k\) for \(H^1(\hat{e})\) and \({\mathbf{Q}_l(\hat{e})}\) of degree \(l\) for \(H(\text{div},\hat{e})\) case.
Correa M. , Rodriguez J. C. , Farias A., de Siqueira D. and Devloo P.R.B. . Hierarchical high order finite element spaces in \(H(\text{div},\Omega) \times H^1(\Omega)\) for a stabilized mixed formulation of Darcy problem. Computers and Mathematics with Applications, 80. (2020) 1117-1141.
Solin P., Segeth K. and Dolezel I.. Higher-Order Finite Elements Methods. Chapman & Hall. 2004.
Boffi D., Brezzi F. and Fortin M., Mixed Finite Element Methods and Applications. Springer Series. 2013.
A reference manual documenting the set of state variables and material properties expected by the plugin, along with all of its supported configuration and result options can be found here.
 1.8.15
1.8.15